Fibonacci Extensions

Learn Forex Trading

Leonardo Bigollo Pisano, also known as Fibonacci, is considered one of the greatest mathematicians in Europe of the Middle Ages (476 AD – 1453 AD). His extensive travels in the Mediterranean as a child with his merchant father exposed him to many different techniques of arithmetic and accounting. Although he laid the groundwork for commercial arithmetic and financial mathematics, he is nowadays mostly known for the Fibonacci numbers and sequence.

In his book Liber Abaci (Book of Calculation) he considered how many rabbits could be produced in a year if a pair is placed in a cage and each pair gives birth to a new pair every month (with the exception that each pair can only breed for the first time after two months). The calculation of the above question led to the Fibonacci sequence.

Fibonacci Sequence

The sequence is derived by adding the preceding two numbers together to get the next one:

To get the next Fibonacci number in the sequence, add 233 to 377 for 610.

What is significant about this pattern is that the ratio of any number to the one before it in the sequence tends to be 1.618. This number is popularly known as the golden ratio and denoted by the Greek letter φ.

Geometry

In geometry, there exists a point on a straight line that:

a/b=a+b/a = φ = 1.618

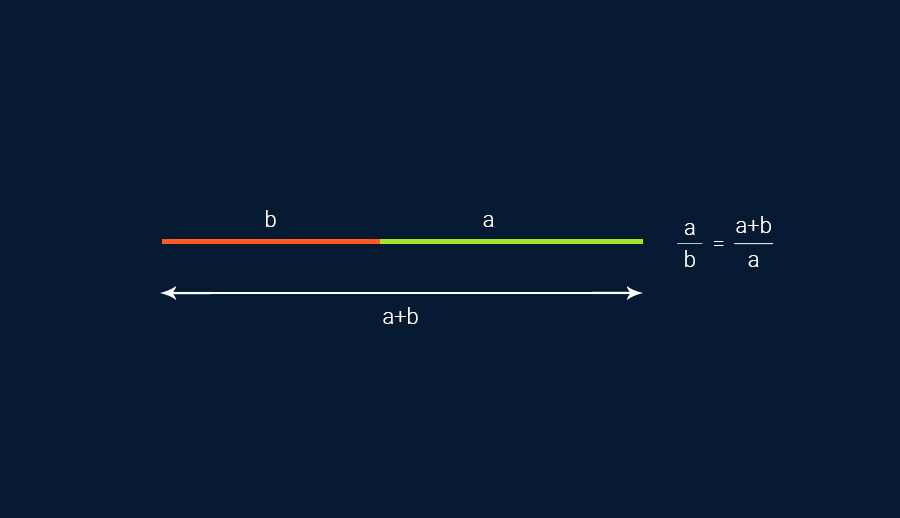
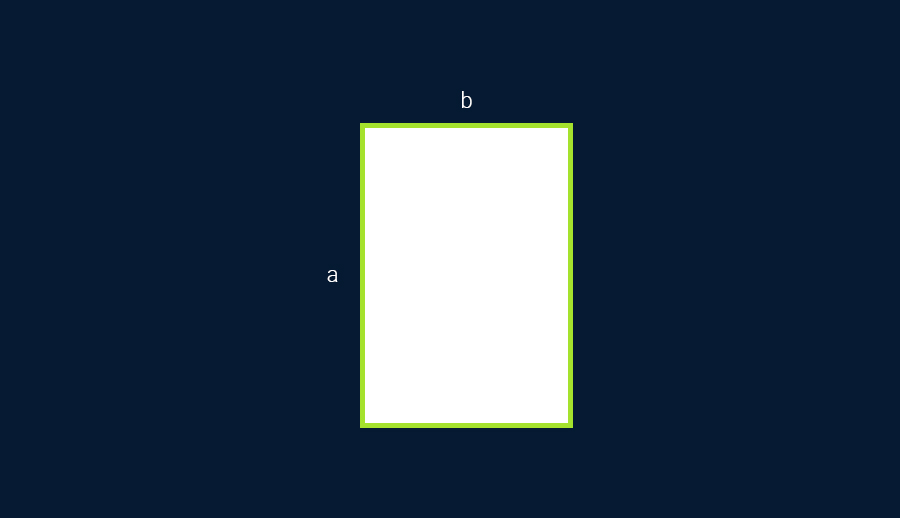
Similarly, the ratio exists in golden rectangles with side a (long side) and b (short side):

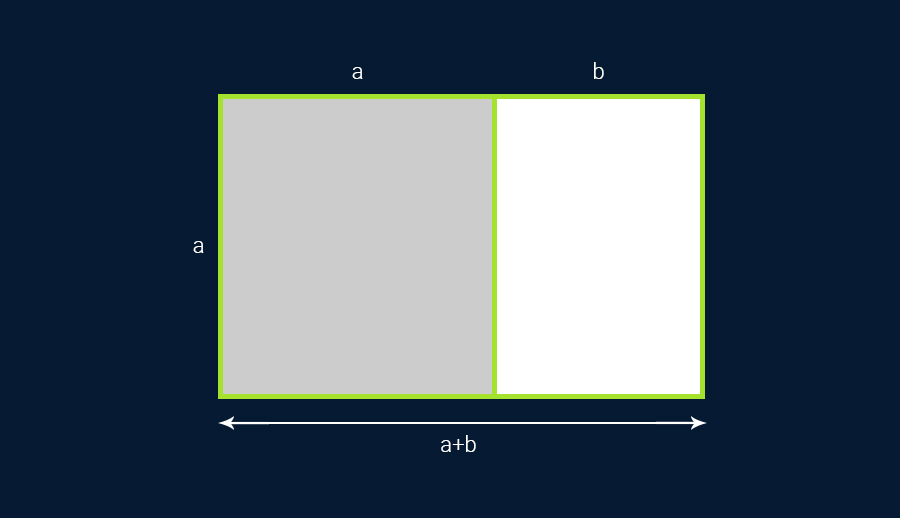
When placed next to a square with sides a, the ratio of the longest side (a+b) to the shortest side (b) is the same as the ratio of the longer rectangle side b to the shortest side b which is equal to the golden mean (1.618).

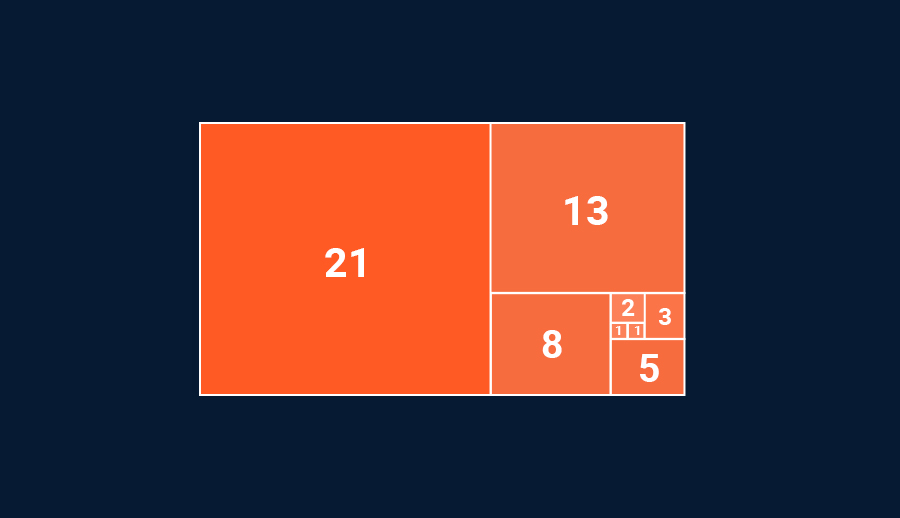
Similarly, the Fibonacci rectangle consists of squares whose sides are Fibonacci numbers.

Architecture

The golden ratio (also known as the golden section or golden mean) appears not only in geometry but also in architecture. The ratio of length to width that approximates 1.618 was considered to be more pleasing to the eye according to ancient Greeks, including the Greek sculptor Phidias.

Mathematics

In mathematics, the golden ratio has the following unique properties:

1/Φ +1=Φ=1/(Φ+1)
Φ2 =Φ+1
Φ2 – Φ -1 =0 (solve the equation to find φ=1+sqrt(5) / 2)

Nature

Surprisingly enough, flowers and plants follow the Fibonacci sequence as well. For example, the mariposa lily has three petals,

while buttercups have five shiny yellow petals.

The sequence continues with examples of flowers with 8, 13, 21, 34 petals and so on.

Human Body

It is also present in the human body. For instance, the width of the central incisor compared to the lateral incisor is in golden ratio.

Fibonacci Extensions

As we have seen, dividing a number in the sequence by the preceding one will result in 1.618. Additionally, dividing a number in the sequence by the number two places lower will result in 2.618. Furthermore, dividing a number in the sequence by a number three places lower will result in 4.236. These ratios are also known as Fibonacci extensions.

Financial Markets

The financial markets and trading are no exception to the prevalence of Fibonacci ratios. Fibonacci ratios, or more specifically extensions, can be used to help estimate potential price targets and take profit and stop loss levels.

For example, by applying the Fibonacci tool on the swing-top and dragging down to the swing-bottom, three price targets may be calculated: 1.618, 2.618 and 4.236. These levels will be potential targets to the upside.

Conversely, by applying the Fibonacci tool on a swing to the downside, three potential profit targets may also be calculated. The Fibonacci tool is attached on the swing-bottom and then dragged to the swing-top to calculate the corresponding price targets: 1.618, 2.618 and 4.236.

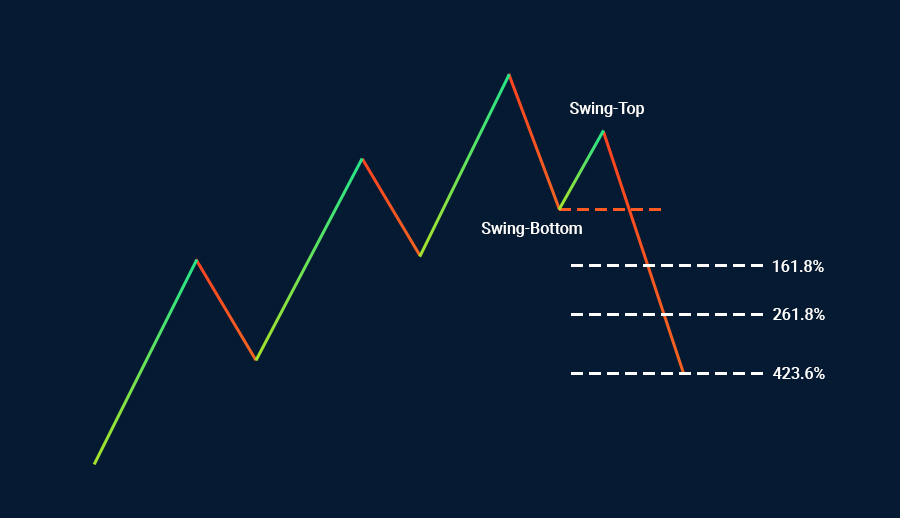
Stop Loss

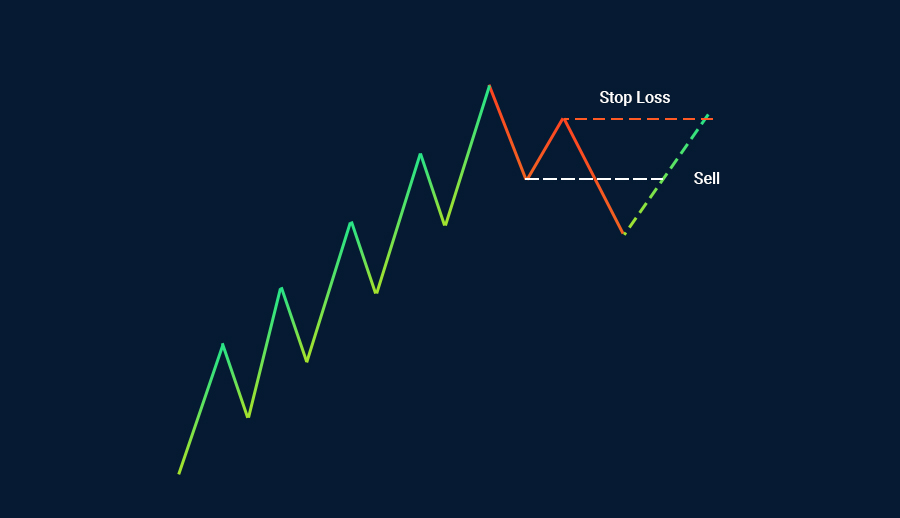
Speaking of Fibonacci take profit levels, one should never forget that the markets don’t always move in the expected direction. Sometimes they move in the opposite direction and so traders should mitigate the risk of losing money by placing a protective stop loss. This way, the risk of the loss of money will be precalculated in advance. For example, after a buy one would expect the market to rise higher. That, of course, is not always the case. Experienced traders know that very well, and that’s why they place a protective stop loss just in case the unexpected happens.

Similarly, after a sell traders should be aware that nothing is 100% certain in the markets and thus a stop loss is highly recommended to mitigate the risk of losing money.

Elliott Wave

Fibonacci extensions are also an important principle for the popular Elliott Wave theory. As you may recall, according to Elliott the markets move in five waves.

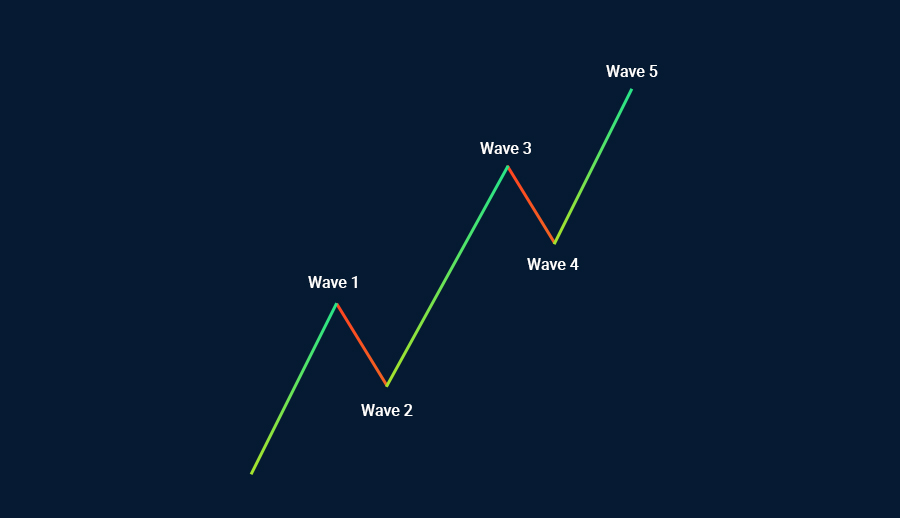
Wave 3 may exist in a ratio of approximately 1.618, 2.618 or 4.236 to Wave 1. This is actually the wave the most traders are after. Why? Simply because based on the theory it is never the shortest and is usually the longest wave among waves 1, 3 and 5.

Conclusion

Fibonacci numbers and their corresponding ratios exist everywhere in life, from mathematics to nature, from architecture to the human body. Even though some may call the existence of these ratios a coincidence, it is an acceptable practice by at least a group of traders to use Fibonacci extensions in estimating potential price and profit or loss targets.

Learn to trade with FXTM

Discover how to make the right trading decisions for your style and goals with our comprehensive range of educational resources. Learn from home when and how it suits you with our educational videos or sign up for a remote webinar. We also host on-location, interactive forex seminars and workshops around the world – there might be one coming to your area soon!